So how might resonant memory work? Here’s a highly simplified diagram:
There are two neurons here, creating a loop. How likely is this to happen with millions of nerve cells spreading dendrites all over the cortex?
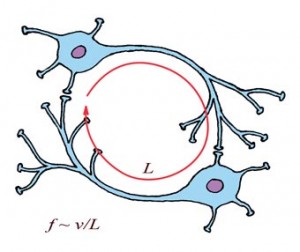
To the extent that it does happen, it could set up a loop with a specific frequency:
The frequency (f) of this loop is approximately equal to the nerve impulse velocity (v) divided by the length of the loop (L). This doesn’t take into account the time added by each synapse, but it’s a rough start. Whatever the velocities of each axon and the lag of each synapse, this particular little loop has a cycle time associated with it. If you hit it with an input with a matching cycle time, this loop will resonate:
The input stream has pulses separated by t, the cycle time of the loop. The first pulse to hit will circle the loop and feed back into the starting neuron, just as the second pulse is coming in. Because the body of the neuron sums the inputs, it will keep sending a strong signal through the loop. The synapses are strengthened when a signal continues to impact them, so this circuit starts to provide stronger and stronger resonant signals as time goes on. We are training this loop to respond to the input.
The output is just the same as the input here, so we haven’t really accomplished much. But we have seen just how easy it is to create a hypothetical neural circuit that can resonate with a given input.